and the distribution of digital products.
DM Television
How 3D-Printed Metamaterials Could Revolutionize Noise Reduction
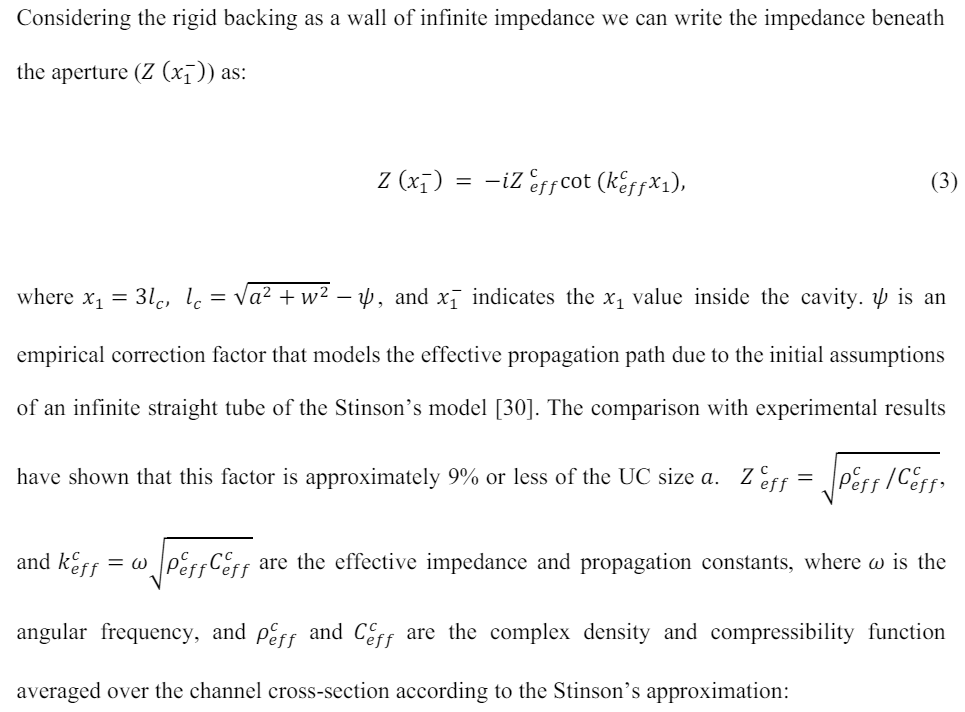
2 Unit cell design and analysis
3 Unit cell experimental and numerical characterization
4 Rainbow AM labyrinthine panel
4.1 Panel design and fabrication
4.4 AM panel sound absorption results
5 Numerical evaluation of different labyrinthine sound absorption panel solutions
5.1 Macrocell with backing cavity
Conclusions, Acknowledgements, and References
ConclusionsIn this work, we have provided an experimental proof of concept for a novel approach to noise attenuation exploiting a rainbow-based design using labyrinthine metamaterials and combining UCs of varying thickness and lateral size in a quasi-periodic arrangement that ensures good homogeneity in the panel response. We have described the full design and validation procedure, from numerical design and modeling of the UC to its characterization in an impedance tube, to the design of the macrocells composing the panel, and its realization using selective laser sintering. The final structure has then been characterized experimentally in a small-scale reverberation chamber, demonstrating a close to ideal absorption over the targeted low frequency range, centred at 1 kHz, thus validating the approach. Finally, detailed FE simulations have allowed to evaluate possible improvements/modifications to the panel by adding a foam filling and a foam backing cavity. The proposed prototype can be further developed, and thanks to its modular design, can be employed in diverse applications, e.g. in room acoustics, in automotive parts, or aeronautics in general. This approach, together with other metamaterial-based solutions proposed in the literature, contributes to an alternative (or complementary) route to the use of traditional sound absorbing materials in noise control. The proposed solution can be particularly attractive due to the reduced thickness of the panels and relatively small density of the required parts emerging from the subwavelength nature of the labyrinthine metamaterials used in the design.
AcknowledgementsFN, VHK, LB, EM, DP, MZ, ASG, LS, FB thank the Alta Scuola Politecnica project “MetaMAPP”. FN, ASG, NMP and FB are supported by the EU H2020 FET Open “Boheme” grant No. 863179.
References[1] G. Ma and P. Sheng, Acoustic Metamaterials: From Local Resonances to Broad Horizons, Science Advances 2, e1501595 (2016).
\ [2] B. Assouar, B. Liang, Y. Wu, Y. Li, J. C. Cheng, and Y. Jing, Acoustic Metasurfaces, Nature Reviews Materials 3, 460–472 (2018).
\ [3] H. Ge, M. Yang, C. Ma, M. H. Lu, Y. F. Chen, N. Fang, and P. Sheng, Breaking the Barriers: Advances in Acoustic Functional Materials, National Science Review 5, 159–182 (2018).
\ [4] X. Zhang, Z. Qu, and H. Wang, Engineering Acoustic Metamaterials for Sound Absorption: From Uniform to Gradient Structures, iScience 23, 101110 (2020).
\ [5] S. Kumar and H. Lee, The Present and Future Role of Acoustic Metamaterials for Architectural and Urban Noise Mitigations, Acoustics 1, 590-607 (2019).
\ [6] J. Li, X. Wen, and P. Sheng, Acoustic Metamaterials, J Appl Phys 129, 171103 (2021).
\ [7] F. Langfeldt and W. Gleine, Improved Sound Transmission Loss of Glass Wool with Acoustic Metamaterials, in Proceedings of the 26th International Congress on Sound and Vibration, ICSV 2019 (2019).
\ [8] G. Palma, H. Mao, L. Burghignoli, P. Göransson, and U. Iemma, Acoustic Metamaterials in Aeronautics, Applied Sciences, 8(6), 971 (2018).
\ [9] M. Yang and P. Sheng, Sound Absorption Structures: From Porous Media to Acoustic Metamaterials, Annual Review Material Research 47, 83–114 (2017).
\ [10] L. Zhao and S. Zhou, Compact Acoustic Rainbow Trapping in a Bioinspired Spiral Array of Graded Locally Resonant Metamaterials, Sensors 19, 788 (2019).
\ [11] M. Yang, G. Ma, Z. Yang, and P. Sheng, Coupled Membranes with Doubly Negative Mass Density and Bulk Modulus, Physical Review Letters 110, 134301 (2013).
\ [12] G. Ma, M. Yang, S. Xiao, Z. Yang, and P. Sheng, Acoustic Metasurface with Hybrid Resonances, Nature Materials 13, 873–878 (2014).
\ [13] V. Romero-García, G. Theocharis, O. Richoux, and V. Pagneux, Use of Complex Frequency Plane to Design Broadband and Sub-Wavelength Absorbers, Journal of the Acoustical Society of America 139, 3395–3403 (2016).
\ [14] N. Jiménez, W. Huang, V. Romero-García, V. Pagneux, and J. P. Groby, Ultra-Thin Metamaterial for Perfect and Quasi-Omnidirectional Sound Absorption, Applied Physics Letters 109, 121902 (2016).
\ [15] V. Romero-Garciá, G. Theocharis, O. Richoux, A. Merkel, V. Tournat, and V. Pagneux, Perfect and Broadband Acoustic Absorption by Critically Coupled Sub-Wavelength Resonators, Scientific Reports 6, 19519 (2016)
\ [16] N. Jiménez, V. Romero-García, V. Pagneux, and J.-P. Groby, Rainbow-Trapping Absorbers: Broadband, Perfect and Asymmetric Sound Absorption by Subwavelength Panels for Transmission Problems, Scientific Reports 7, 13595 (2017).
\ [17] J. Boulvert, J. Costa-Baptista, T. Cavalieri, V. Romero-García, G. Gabard, E. R. Fotsing, A. Ross, M. Perna, J. Mardjono, and J.-P. Groby, Folded Metaporous Material for Sub-Wavelength and Broadband Perfect Sound Absorption, Applied Physics Letters 117, 251902 (2020).
\ [18] J. Boulvert, T. Humbert, V. Romero-García, G. Gabard, E. R. Fotsing, A. Ross, J. Mardjono, and J.-P. Groby, Perfect, Broadband, and Sub-Wavelength Absorption with Asymmetric Absorbers: Realization for Duct Acoustics with 3D Printed Porous Resonators, Journal of Sound and Vibration 523, 116687 (2022).
\ [19] Z. Liang and J. Li, Extreme Acoustic Metamaterial by Coiling up Space, Physical Review Letters 108, 114301 (2012).
\ [20] Z. Liang, T. Feng, S. Lok, F. Liu, K. B. Ng, C. H. Chan, J. Wang, S. Han, S. Lee, and J. Li, SpaceCoiling Metamaterials with Double Negativity and Conical Dispersion, Scientific Reports 3, 1614 (2013).
\ [21] Y. Xie, A. Konneker, B. I. Popa, and S. A. Cummer, Tapered Labyrinthine Acoustic Metamaterials for Broadband Impedance Matching, Applied Physics Letters 103, 201906 (2013)
\ [22] S. K. Maurya, A. Pandey, S. Shukla, and S. Saxena, Double Negativity in 3D Space Coiling Metamaterials, Scientific Reports 6, 33683 (2016)
\ [23] Y. Xie, B. I. Popa, L. Zigoneanu, and S. A. Cummer, Measurement of a Broadband Negative Index with Space-Coiling Acoustic Metamaterials, Physical Review Letters 110, 175501 (2013).
\ [24] Y. Liu, W. Xu, M. Chen, T. Yang, K. Wang, X. Huang, H. Jiang, and Y. Wang, Three-Dimensional Fractal Structure with Double Negative and Density-near-Zero Properties on a Subwavelength Scale, Materials & Design 188, 108470 (2020).
\ [25] G. Y. Song, Q. Cheng, B. Huang, H. Y. Dong, and T. J. Cui, Broadband Fractal Acoustic Metamaterials for Low-Frequency Sound Attenuation, Applied Physics Letters 109, 131901 (2016).
\ [26] C. Zhang and X. Hu, Three-Dimensional Single-Port Labyrinthine Acoustic Metamaterial: Perfect Absorption with Large Bandwidth and Tunability, Physical Review Applied 6, (2016).
\ [27] A. O. Krushynska, F. Bosia, M. Miniaci, and N. M. Pugno, Spider Web-Structured Labyrinthine Acoustic Metamaterials for Low-Frequency Sound Control, New Journal of Physics (2017).
\ [28] A. O. Krushynska, F. Bosia, and N. M. Pugno, Labyrinthine Acoustic Metamaterials with SpaceCoiling Channels for Low-Frequency Sound Control, Acta Acustica United with Acustica 104, 200-210 (2018).
\ [29] M. Molerón, M. Serra-Garcia, and C. Daraio, Visco-Thermal Effects in Acoustic Metamaterials: From Total Transmission to Total Reflection and High Absorption, New Journal of Physics 18, (2016).
\ [30] Foam Core Impresses in Aircraft Study, Reinforced Plastics 58, 31-33 (2014).
\ [31] S. Ghinet, P. Bouche, T. Padois, L. Pires, O. Doutres, T. C. Kone, K. Triki, F. Abdelkader, R. Panneton, and N. Atalla, Experimental Validation of Acoustic Metamaterials Noise Attenuation Performance for Aircraft Cabin Applications, in Proceedings of 2020 International Congress on Noise Control Engineering, INTER-NOISE 2020 (2020).
\ [32] T. Cox and P. d’Antonio, Acoustic Absorbers and Diffusers: Theory, Design and Application (CRC press, 2016).
\ [33] N. Jiménez, O. Umnova, and J.-P. Groby, Acoustic Waves in Periodic Structures, Metamaterials, and Porous Media, Ch. “The Transfer Matrix Method in Acoustics”, Springer International Publishing, (2021).
\ [34] Vincent Laude, Phononic Crystals (de Gruyter, 2020).
\ [35] V. Romero-García, G. Theocharis, O. Richoux, and V. Pagneux, Use of Complex Frequency Plane to Design Broadband and Sub-Wavelength Absorbers, Journal of the Acoustical Society of America 139, 3395 (2016).
\ [36] A. O. Krushynska, F. Bosia, and N. M. Pugno, Labyrinthine Acoustic Metamaterials with SpaceCoiling Channels for Low-Frequency Sound Control, Acta Acustica United with Acustica 104, (2018).
\ [37] A. Magnani, C. Marescotti, and F. Pompoli, Acoustic Absorption Modeling of Single and Multiple Coiled-up Resonators, Applied Acoustics 186, 108504 (2022).
\ [38] Y. Wang, H. Zhao, H. Yang, J. Zhong, D. Zhao, Z. Lu, and J. Wen, A Tunable Sound-Absorbing Metamaterial Based on Coiled-up Space, Journal of Applied Physics 123, 185109 (2018).
\ [39] G. do N. Almeida, E. F. Vergara, L. R. Barbosa, and R. Brum, Low-Frequency Sound Absorption of a Metamaterial with Symmetrical-Coiled-up Spaces, Applied Acoustics 172, 107593 (2021).
\ [40] M. R. Stinson, The Propagation of Plane Sound Waves in Narrow and Wide Circular Tubes, and Generalization to Uniform Tubes of Arbitrary Cross‐sectional Shape, Journal of the Acoustical Society of America 89, 550 (1991).
\ [41] ISO 354, Acoustics — Measurement of Sound Absorption in a Reverberation Room, International Standard (2003).
\ [42] ASTM, Standard Test Method for Impedance and Absorption of Acoustical Materials Using a Tube, Two Microphones and a Digital Frequency Analysis System, American Society for Testing of Materials (1990).
\ [43] ASTM E2611, Standard Test Method for Normal Incidence Determination of Porous Material Acoustical Properties Based on the Transfer Matrix Method E2611, American Society for Testing of Materials (2019).
\ [44] D. Pilon, R. Panneton, and F. Sgard, Behavioral Criterion Quantifying the Effects of Circumferential Air Gaps on Porous Materials in the Standing Wave Tube, Journal of the Acoustical Society of America 116, 344–356 (2004).
\ [45] M. R. Schroeder, The ‘“Schroeder Frequency”’ Revisited, Journal of the Acoustical Society of America 99, 3240–3241 (1996).
\ [46] G. Kirchhoff, Ueber Den Einfluss Der Wärmeleitung in Einem Gase Auf Die Schallbewegung, Annalen der Physik 210, 177 (1868).
\ [47] C. Zwikker and C. W. Kosten, Sound Absorbing Materials, Elsevier (1949).
\ [48] N. Atalla and F. Sgard, Modeling of Perforated Plates and Screens Using Rigid Frame Porous Models, Journal of Sound and Vibration 303, 195 (2007).
\
Appendix IThe general theory of sound propagation in a cylindric tube in presence of viscous-thermal losses was initially developed by Kirchhoff [46] using the linearized Navier-Stokes equation. Given the complexity of the analytical solution, Zwikker and Kosten [47] developed approximate solutions for narrow and wide tube diameters. Subsequently, Stinson [40] derived an approximate solution for an infinitely long tube with arbitrary cross-sectional shape. This model was employed to calculate the variation of impedance along the coiled-up tube (Fig. 1b). The thin inlet was instead described using the Johnson-Champoux-Allard model (JCA), as pointed out in [48]. The model allows to include not only the losses taking place along the inlet, but also those around the aperture due to the sudden cross-section variation.
\
\
\
:::info This paper is available on arxiv under CC BY-NC-ND 4.0 DEED license.
:::
:::info Authors:
(1) F. Nistri, Department of Applied Science and Technology, Politecnico di Torino, Torino, Italy and Politecnico di Milano, Milano, Italy;
(2) V. H. Kamrul, Politecnico di Milano, Milano, Italy;
(3) L. Bettini, Politecnico di Milano, Milano, Italy;
(4) E. Musso, Politecnico di Milano, Milano, Italy;
(5) D. Piciucco, Politecnico di Milano, Milano, Italy;
(6) M. Zemello, Politecnico di Milano, Milano, Italy;
(7) A.S. Gliozzi, Department of Applied Science and Technology, Politecnico di Torino, Torino, Italy;
(8) A.O. Krushynska, Faculty of Science and Engineering, University of Groningen, Groningen, The Netherlands;
(9) N. M. Pugno, Laboratory for Bioinspired, Bionic, Nano, Meta Materials & Mechanic, University of Trento, Trento, Italy and School of Engineering and Materials Science, Queen Mary University of London, United Kingdom;
(10) L. Sangiuliano, Phononic Vibes s.r.l., Milano, Italy;
(11) L. Shtrepi, Department of Energy "Galileo Ferraris", Politecnico di Torino, Torino, Italy;
(12) F. Bosia, Department of Applied Science and Technology, Politecnico di Torino, Torino, Italy and a Corresponding Author ([email protected]).
:::
\
- Home
- About Us
- Write For Us / Submit Content
- Advertising And Affiliates
- Feeds And Syndication
- Contact Us
- Login
- Privacy
All Rights Reserved. Copyright , Central Coast Communications, Inc.